Lesson #6 - Make Your Own Fibonacci Sequence

Ok, we have come to the final lesson in this journey. Your final task is a little more open-ended, but should be a fun one. I would like you to come up with your own Fibonacci Sequence.

A Triangular Number Sequence
Imagecredit: Math is Fun.com

I know what your first reaction may be: How is that possible? Isn't there only one Fibonacci Sequence?

And indeed, technically you may be correct. But think for a second about what makes this sequence so simple. All you do is add two consecutive numbers in the sequence to find the next number. That's pretty simple, right?

The Fibonacci Sequence (the real one) begins with the numbers 1 and 1. From there, 1+ 1 =2, 1 + 2 = 3, and so on.

A Pentagonal Number Sequence
Image credit: astarmathsandphysics.uk

What I would like you to do is to pick two different numbers to start your sequence. They can be the same numbe
r twice, just like Fibonacci did. Something like 2, 2, perhaps. Or they can be different numbers. Maybe 2, 3. Or you could pick two totally outlandish numbers, like -5 and 7.

It doesn't matter which two you pick. What's most important is that you figure out how to produce the next values in the sequence and then go exploring to see what the sequence holds in store.

Some questions to ponder:

A Hexagonal Number Sequence, of sorts
Imagecredit: drking.org.ca

• What common ratio does your sequence eventually lead to? The Fibonacci Sequence leads to 'phi', or approximately 1.618, which is often called the Golden Ratio. Does your sequence approach a similarly small value? Is it close to 'phi'?
• Is there any way to create a sequence of numbers that eventually matches the original Fibonacci numbers, if not all, at least some? 
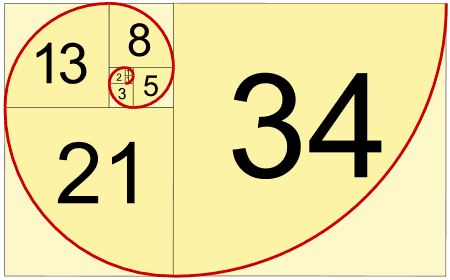
• Is it possible to produce a spiral like the one the Fibonacci Sequence produces that could possibly be mirrored in nature?

This is the time to use your creativity and see where it takes you. Who knows? Maybe you could tell a story like Fibonacci did about rabbits that leads to a powerful new number pattern. And then, just maybe, in another thousand years (or less) math students of the future could find themselves studying the (Your Name Here) Sequence.

Good luck - good math!

-Tom Robinson

Pascal's Triangle (Stay tuned for Pascal's Orchard!)
Image credit: MtDouglas.ca

Here is a link to a Math Is Fun site that explores other number sequences you may be interested in. They are not Fibonacci sequences, but might be fun to discover on your own.

Lucas Numbers

I came across this video by chance, though I love the Numberphile guys (and gals) and their work. It explains the concept of Lucas numbers, which are directly related to Fibonacci numbers, but are pretty intriguing in an unexpected way. I won't spoil the fun, but do take some time to watch - you will be surprised by what you learn, though I do take exception to his claim that Lucas Numbers are actually better than Fibonacci. Call me biased...

Lucas Numbers - from Numberphile's YouTube Channel 

featuring Matt Parker

Lesson #5 - Creating Integers From Fibonacci numbers

Objective: Students will use Fibonacci numbers and the four arithmetic operations to produce integer values from 1-20, 1-50, 1-100.

Photo credit:
DevCentral

Task: Using only Fibonacci Sequence numbers and the four arithmetic operations (addition, subtraction, multiplication and division) produce each integer value from 1-20. Older students may be challenged to produce numbers through 50, or even 100.

Example: The number 10 may be produced in the following ways using Fibonacci numbers:
5+5
8+2

Photo credit:
MathIsFun.com

13 - 3

The number 17 may be produced by doing the following:
13 + 5 - 1
8*3-13 + 3*2

• How many different ways can you find to make each target number?
• Which numbers can you find using only addition?
• Which numbers can you find using only addition and subtraction without repeating any numbers?
• Which numbers can you find using all four operations?